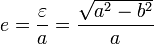Kepler

What does the 3rd Kepler’s law say?

Hi,
today I want to do a short post about the 3rd Kepler’s law. I kind of really like it because it has very simple explanation but lot of uses at the same time.

The law goes as follow:{\frac {T_{1}^{2}}{T_{2}^{2}}}={\frac {a_{1}^{3}}{a_{2}^{3}}}

T stands for time and a for semi-major axis of ellipse, that is basicly radius for planets since

What is semi-latus rectum?

their orbit is highly circular. The index 1 and 2 stands for first and second object, basicly you are comparing two objects with each other though they must orbit the same body. This is very useful since you can compare anything in Solar System orbiting Sun with Earth. Why is it useful? Because Earth’s semi-major axis is 1AU and orbit lasts for 1 year which means that this fraction will disappear and you are left only with the object you want to calculate with.

Where did this even came from? The prove for this equation is very simple and basicly stands on the fact that centripetal force equals gravitational force for our orbiting object.

Fg=Fc

We can find the equations for both of these forces and from them finally get to the Kepler’s law:KeplerLaw3

Ok, before you start to freak out, this is completely easy. First line is clear, I have accidentaly indexed Fd instead of Fc because in Czech the force is called “dostředivá”.

Second line shows the forces and their equations, third canceles the mass of the orbiting body and the radius of orbit. Since v=s/t we can write it down as is shown. Also watch out because s is whole orbit so s^2=4π^2

The equation that you have in fifth and six line is also usable equation! It is more general and does not need the second orbiting body but it needs the mass of object. From this equation you can also figure out the mass of Sun which is completely amazing! (You have to watch out for the right units!)

After the small space I have divided the equation by the same one except that it works with some other object orbiting the same star (or planet..), with this step I will get easily rid of all the π, gravitational constant and mass of the center object.

Now we have the original 3rd Kepler’s law!

Dragallur

PS: in the prove we also assumed that r=a which means that planets orbit on circles not ellipses but it is accurate enough

What is going up on Mars?

Hi,
while thinking about today’s topic I find some random stuff and decided to share it with you. So here you go, new exoplanets, tsunami on Mars, good view on Mars and SpaceX announcement.

Artist’s impression of exoplanet.

Thanks to new statistical method there were 1,284 exoplanets confirmed[1].
This is biggest number ever in one shot. There is of course lot of data form Kepler which observes stars for those exoplanets. You can not be sure always if what you saw was really exoplanet so you need more data. When uncertainity is below 1%, it is officially agreed that the planet is there. With this new method, lot of those uncertainities went below 1% so yes they were “discovered”. Other data was “thrown away” because the percentage went lower and generally this was nice breakthrough.[2]

For long time it was thought that Mars’s terrain was shaped by its ocean that later on evaporated. But the shoreline would be graduall which is not what we observe, rather exact opposite with many geological features along it. These features could rather be explained by huge tsunamis that were formed by asteroid impacts.

Also Mars is going to be these weeks in oposition to Sun and basically really close to Earth, on about 1/2 of AU. The closest it could be would be about 1/3 of AU because its orbit is kind of excentric. If you want to see it well, just take a telescope and with good one you should be able to see even some of its geological features. If you get to see (if it is possible for normal people) Olympus Mons, say hello from me 😉

After SpaceX landed second time on the barge, Elon Musk stated that they want to land on Mars in the year of 2018, which is in two years! They have really lot of work to do so lets see where they will be in that time! (Read more here on the blog of Phil Plait)

Dragallur

[1]Exoplanets are planets that orbit around other stars.
[2]From the data we also know that 550 are probably rocky and 21 are in habitable zone.

When astrology was a real thing

Hi,
it is important to distinquish the difference between astrology and astronomy. The most basic is that nowadays astrology is no longer science field.

Today I want to talk about astrology when it was mainstream. For this to be true we have to move back few years, I want talk about renessaince, so that is about 400 years.

These days astronomy has gained a great improvement since old times. This improvement is mostly in two things: heliocentric model and first telescope.

There were couple of important people who all worked on this heliocentrism, it whole started with Copernicus, followed by Tycho Brahe, Johannes Kepler and Galileo Galilei. Now I want talk about Tycho and Johannes because both of them were astrologers too.

Tycho Brahe

Tycho Brahe is known as one of the most or simply most precise observer. It is said that he was able to make measurements 10 times more accurate then most other people (means measurement of 1/4 of arcminute). He probably has the least credit for heliocentrims of those four guys because at one point he rejected the idea and rather made his own geo-helio centric model.

Anyway he was working on the court of Rudolf II. The Holy Roman Emperor. Not only that he was making very good measurements but he also created zodiac for the king.

While there are tons and tons of zodiacs, usually it is about the position of celestial objects. Imagine clock laid on the ecliptic. Then switch all  the numbers for the signs in zodiac. Then when planet passes in the angle which is for the zodiac you say that for example: “Mars is in the sign of Pisces”. Those are also actuall constellations on sky.

Zodiac with constellations

Johannes Kepler

Johannes Kepler is known for his laws of planetary motion. He did not have very good eyesight so when he met with Tycho Brahe he just helped him with math and also with processing all the data that he measured, though Tycho guarded them carefully.

Kepler was also interested in astrology, actually he probably spent the same amount of time on astronomy as on astrology. He worked with Tycho also on the court of Rudolf II. who was very willing to finance their exploration.

 

It took some more years for people to realise that as astrology also alchemy is not a science and is not worth of time.

Dragallur

To read more check out these pages: 1) 2) 3) 4)

Also check out this cool blog about math!

Easy way to get excentricity

Hi,
it has been few days since I looked on some problems from astronomy contest. One of the problem was about excentricity of Pluto.

This has to do with ellipses since according to Kepler’s laws, planets are orbiting on ellipses, shapes just a little different from circles, at least when you consider their equation.

For circle equation is x2+y2=1

For ellipse equation is x2/a+y2/b=1

Sun is always the focus of the elipse, above those two points are -c,0 and c,0. For planets those ellipses are much less excentric which means that in the equation above, “a” and “b” are fairly similar.

In the problem I knew only perihelium and afelium of Pluto.

e = \frac{\varepsilon}{a}=\frac{\sqrt{a^2-b^2}}{a}

“e” is excentricity. “a” is semi-major axis. “b” is semi-minor axis. “ε” is linear excentricity (not really important).

Since the equation goes as the one above you need both semi-major and semi-minor axis to get the result. From knowing afelium and perihelium I easily got semi-major axis. To get semi-minor you must know that the distance from focal point to the top or bottom of ellipse is equal to semi-major axis, from this you can use pythagorean theorem and then all this information insert into the equation. All went right and with perihelium of 29.66 AU and afelium of 49.32 AU the excentricity is 0.246 which is just right, if excentricity is equal to 1 than it is parabola and if greater it is hyperbola.

Dragallur

Transit photometry

Kepler 186f

Hi,
Today I will write about transit photometry.
This is method of finding exoplanets (means those which are not in our Solar System).

Of course we can not see any of those planets because they are too small. But what we can see or better what can Kepler Space Telescope see is effect of such a exoplanet when it transits around its star. To see this clearly, watch this video.

To describe it with words, when planet transits (orbits) star and Kepler is watching it he will see the little difference in its brightness, some of the light will be blocked by some planet. When this happens periodically we know that there is some planet.
Of course this has some limitations, we can only see objects that are orbiting close to its star because no telescope or satellite has service life of 30 years which would be needed
for Saturn if some extraterrestrial life would watch Sun. Those 30 years are counting with Saturn transiting Sun at the time of telescope´s start.

So this is transit photometry which can be used only for enough large planets that are not really far away from their stars. Usually scientist are mostly trying to find planets in “life zones”. Those zones are in some fixed distance depending on the type of star and it means that we think there could be live. There is lot of candidates for habitable planets and probably best of them is Kepler 452b which is orbiting G type star (same as Sun). Kepler Small Habitable Zone Dozen
Because legendary Kepler is getting old and he is able to watch only fixed part of sky there is TESS (Transiting Exoplanet Survey Satellite) which will be able to watch 90%.

In the year of 2024 there will also come PLATO (Planetary Transits and Oscillations of stars). This mission is planned for six years but still it will no be able to find planets with the size of Mars or Mercury.

Last one is NGTS (Next Generation Transit Survey) which is trying to find planets smaller than Neptune around stars with apparent magnitude lower than 13 (7 is not visible to average human eye).

Also astronomers can find out how big is exoplanet by its gravitational effect on star and from this we can even count what is its density.

Dragallur